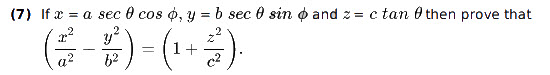Grade 10 IMO – Tuyển chọn câu hỏi luyện thi IMO Lớp 10

Grade 10 IMO

Week 1

Choose correct answer(s) from the given choices

(1) If cosec θ − sin θ = m and sec θ − cos θ = n, then the value of {\left( {{m^2}n}\right)^{\frac{2}{3}}} + {\left( {m{n^2}} \right)^{\frac{2}{3}}} is

a. 0        b. 2
c. 1        d. None of these

(2) Find the points of intersection of the parabola y = x² − 7x + 32 and the line y = 4x + 2.

a. (5, 26) and (6, 22)
b. (26, 5) and (22, 6)
c. (5, 22) and (6, 26)
d. (5, 6) and (22, 26)

(3) From a tower on a straight road, the angles of depression of two cars at an instant are 45° and 60°. If the cars are 10 m apart, find the height of the tower.

a. 7\left( {1 + \sqrt 2 } \right)
b. 5\sqrt 2
c. 5\left( {3 + \sqrt 3 } \right)
d. 9\left( {1 + \sqrt 2 } \right)

(4) Let S be the smallest positive multiple of 15 that comprises exactly digits with k ‘0’s, k ‘3’s and k ‘8’s. Find the remainder when S is divided by 8

a. 9            b. 6
c. 0            d. 8

(5) A man on the deck of a ship, 22 meters above the water level, observes that the angle of elevation of the top of a cliff is 60∘ and the angle of depression of the base of the cliff is 45∘ . Find the height of the cliff.

a. 38.1 meters        b. 120.2 meters
c. 60.1 meters        d. None of these

Fill in the blanks

(6) A gymnasium weighs all the people that come in to exercise. On one morning, it notes down the weight of 7 people in kgs as follows: 60 kg, 66 kg, 75 kg, 70.1 kg, 69 kg, 65.9 kg, 84 kg.

The average of their weights is calculated but then one more person comes in to exercise.The new average is calculated to be 71.7 kg then the weight of the new person is ………….. kg.

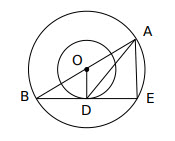
(7) In the given figure, the radii of two concentric circles are 10cm and 5cm. AB is the diameter of the bigger circle and BD is a tangent to the smaller circle touching it at D. . The length of AD is ………. cm.

Answer the questions

(8) It is given that 3 is a zero of the polynomial (x³ – 6x² + 5x + 12), find its other zeros.

(9) The students of a school are made to stand in rows. If the number of students in each row is increased by 6, there would be 4 row less. If the number of students in each rows is reduced by 6, there would be 5 rows more. Find the number of students in the school.

(10) The sum of the first three terms of an AP is 141, and the sum of the next three terms is 339. What is the value of the 10th term?

Week 2

Choose correct answer(s) from the given choices

(1) Find the relation between x and y such that the point P(x,y) is equidistant from the points A(9,5) and B(11,12)

(2) A straight highway leads to the foot of the tower. A man standing at the top of the tower observes a car at an angle of depression of 30∘, which is approaching the foot of the tower with a uniform speed. 7 minutes later, the angle of depression of the car is found to be 60∘. What is the time taken by the car after 7 minutes to reach the foot of the tower?

a. 13.5 minutes      b. 63.5 minutes
c. 3.5 minutes        d. 8.5 minutes

Fill in the blanks

(3) The area of the triangle formed by joining the midpoints of the sides of the triangle whose vertices are A(8,8),  B(4,16) and C(12, 20) is ………… sq.unit.

(4) The sum of all multiples of 4 lying between 211 and 809 is .(The numbers should not be included.)

Answer the questions

(5) Consider a sequence of real numbers {an} defined by

(6) The speed of a boat in still water is 25 km/hour. . It goes 120 km upstream and return downstream to starting point in 10 hour. . Find the speed of the stream.

(8) If cosec θ − cot θ = √2 cosec θ, show that cosec θ + cot θ = √2 cot θ

(9) Simplify (1 + cotθ – cosecθ) (1 + tanθ + secθ)

(10) Three distinct positive integers are such that they differ from each other by at most 6. It is also known that the product of these three integers is 1760. What is the smallest integer among them?

Hits: 1